Gravitational Averaging: A Framework for Directional Gravity in the Cosmic Web

The video displays an abridged podcast version of this paper.

https://youtu.be/D_XyqMT7G4Y?si=ul2jyYX_30_tbsnj

Abstract

Gravity is not uniform but emerges as the weighted average of directional gravitational charges from all “node” or body connections in the universe. Using dark matter filaments as conduits, this model provides a dynamic explanation for local and universal gravitational effects, bridging gaps in current theories (Navarro et al., 1996; Dietrich et al., 2012).

Background

Newtonian Gravity:

• Newton’s law of universal gravitation states that every mass exerts an attractive force on every other mass. This force is proportional to the product of their masses and inversely proportional to the square of the distance between them.
• Mathematically, this is expressed as: where is the gravitational force, is the gravitational constant, and are the masses, and is the distance between their centers.
• Newtonian gravity works well for small-scale systems like planetary orbits but does not account for relativistic effects or anomalies in large-scale cosmic structures.

Einstein’s General Relativity:

• Relativity describes gravity as the curvature of spacetime caused by mass and energy. Objects follow geodesics in this curved spacetime, resulting in what we perceive as gravitational attraction.
• The Einstein field equations: relate the curvature of spacetime to the energy and momentum of matter and radiation .
• General relativity has been highly successful in explaining phenomena like gravitational lensing and the expansion of the universe.

Dark Matter’s Role:

• Observations of galaxy rotation curves and large-scale cosmic structures reveal gravitational effects that cannot be explained by visible matter alone. Simulations like the Millennium Simulation suggest that dark matter forms filaments connecting massive nodes (galaxy clusters), which serve as gravitational highways across the universe (Springel et al., 2005; Forero-Romero et al., 2009).
• Despite its critical role in current models, the nature of dark matter remains unknown, and its integration into gravitational theory is incomplete.

Challenges in Explaining Large-Scale Structure Stability and Dark Matter Distribution

Galactic Stability:

• Galaxy rotation curves remain flat at large radii, suggesting the presence of unseen mass.
• Newtonian and relativistic models struggle to explain this without introducing dark matter.

Cosmic Web:

• The universe’s large-scale structure forms a “cosmic web” of galaxies and filaments, stabilizing over billions of years despite expansion (Bond et al., 1996; Schaye et al., 2015). Current models often fail to explain the interplay between gravitational dynamics and cosmic web stability.

Dark Matter Distribution:

• Simulations like the Millennium Simulation suggest that dark matter forms filaments connecting massive nodes (galaxy clusters).
• How these filaments influence local and universal gravitational effects remains poorly understood.

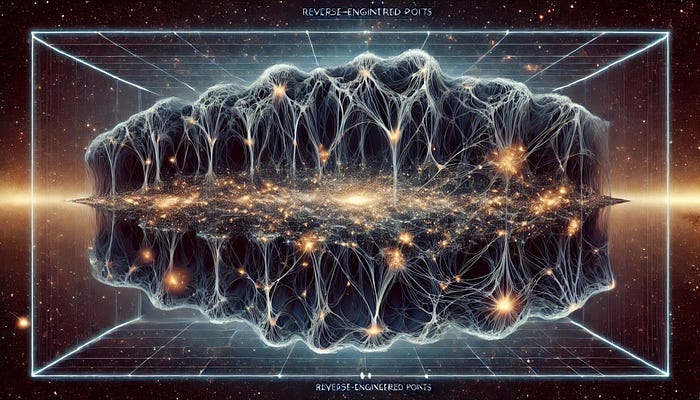
Purpose

Introducing Gravitational Averaging

Gravitational averaging proposes that gravity is not localized but an emergent property of the cumulative gravitational influence of nodes interconnected by dark matter filaments. Filaments act as adaptive, directional pathways transmitting gravitational force, unifying local planetary dynamics with the cosmic web’s stability (Dietrich et al., 2012; Vogelsberger et al., 2014).

The Filament Length Principle

Dark matter filaments act as conduits of gravitational influence. Their length and density determine the strength of the gravitational pull between nodes.

The principle that gravitational influence decreases with filament length and density echoes the inverse-square law and explains tapering effects observed in simulations of cosmic structures (Forero-Romero et al., 2009).

Unifying Local and Universal Effects:

Current models separate local (e.g., planetary orbits) and universal (e.g., galactic stability) gravitational effects. Gravitational averaging provides a unified framework:

• Local gravity emerges from the cumulative effects of nearby nodes.
• Universal stability arises from the directional averaging of filaments across cosmic scales.

Hypothesis and Vision:

By redefining gravity as a networked phenomenon, this model addresses gaps in understanding large-scale structure stability and dark matter’s role.

The gravitational averaging theory could bridge the gap between relativity, dark matter, and quantum scales, offering a more cohesive understanding of the universe.

2. Core Theory

2.1 Central Nodes and Filaments

Defining a Node and Its Central Gravitation Charge

A node represents any mass concentration that exerts and experiences gravitational influence. Examples of nodes include:

• Planets: Earth, Mars, and other celestial bodies within a solar system.
• Stars: Such as the Sun, which dominates the gravitational dynamics of its local system.
• Galaxy Clusters: Massive conglomerations of galaxies interconnected by dark matter filaments.

Each node is characterized by a central gravitational charge, which is the total gravitational influence it exerts due to its mass and its connections to other nodes via dark matter filaments.

The central gravitational charge at a node is influenced by:

• The node’s intrinsic mass (e.g., baryonic matter like stars and planets).
• The additive effect of connected nodes via dark matter filaments, which transmit gravitational influence over vast distances.

Dark Matter Filaments as Directional Pathways

Structure of Filaments:

• Dark matter filaments are elongated structures that act as gravitational highways, connecting nodes across the universe.
• These filaments vary in thickness and density, with denser regions transmitting stronger gravitational influence.

Directional Influence:

• Filaments guide the directionality of gravitational pull between nodes.
• The gravitational force experienced by a node is not uniform but a weighted average of all connected nodes, with filament length and density determining the strength of influence.

The image illustrates how the outward pull of dark matter on a galaxy is like an inverted, cushioned mattress — pulling masses of stars apart and keeping systems from collapsing in on themselves.

Filament Length Principle:

• The gravitational influence decreases with the square of the filament’s length, following the inverse-square law: where is the force transmitted along the filament, is the gravitational constant, is the mass of the connected node, and is the filament length.

Dynamic Adaptation:

• Dark matter filaments adapt dynamically to the mass and connectivity of their nodes, ensuring stability. This self-regulating behavior prevents fragmentation and maintains coherence across scales (Springel et al., 2005; Dolag et al., 2009).

Implications for Stability:

• Local Stability: Gravitational averaging provides insights into planetary orbits and galactic clustering. For example, Earth’s stable orbit reflects the gravitational pull from the Sun, neighboring planets, and the solar system’s dark matter filaments. This dynamic interaction mirrors the stabilization of galaxy clusters by dense, short filaments (Peebles, 1980; Navarro et al., 1996).
• Universal Connectivity: The cosmic web’s interconnected structure ensures that gravitational forces remain balanced across vast scales, stabilizing clusters and superclusters despite the universe’s expansion (Bond et al., 1996; Dietrich et al., 2012).

This video demonstrates the principles of gravitational averaging applied to planetary formation. Using Blender, we simulated multiple spheres with varying masses and initial positions. As the spheres interact dynamically, gravitational pulls and collisions guide the system toward stabilization. Over the course of the simulation, the spheres achieve equilibrium, creating a balanced and predictable configuration reminiscent of natural planetary systems. This visualization highlights the potential of gravitational averaging to model complex cosmic interactions with elegance and efficiency.

2.2 Additive Gravitational Charge

The Principle

The gravitational effect on a body is the cumulative average of all connected nodes.

Purpose:

This formula formalizes the idea that gravitational charge is an emergent property of dark matter filaments connecting nodes in the universe.

Implications:

• It bridges local and universal gravitational effects.
• It shows how gravity arises from the summation of filamentary connections.
• Mathematical expression:

• The total gravitational force on a central node (e.g., a planet, star, or galaxy center).
• This is the vector sum of all gravitational influences acting on the node.

• The summation operator, representing the additive nature of gravitational forces from all N connected nodes in the system.

• The gravitational constant, a scalar that defines the strength of gravity in the system.
• In realistic systems this is

• The mass of the i-th node connected to the central node. Represents how much gravitational charge the node contributes.
• Gravitational influence decreases with the square of the distance.

• The length of the filament or the distance between the central node and the i-th node.
• Gravitational influence decreases with the square of the distance.

• The directional unit vector pointing from the central node to the i-th node.
• This ensures that gravitational forces are calculated as vectors, accounting for directionality.

2.3 Filament Length Principle

Length and Density of Filaments and Gravitational Strength

Filament Length:

• The length of a dark matter filament dictates the gravitational influence it can transmit. Shorter filaments create stronger connections, while longer filaments dilute gravitational effects due to the inverse-square law.
• Nodes connected by shorter filaments experience more direct gravitational pull, creating tightly bound systems like galaxies or solar systems.

Filament Density:

• Filaments with higher density can transmit greater gravitational force over a given length.
• Dense filaments are often observed in regions of high mass concentration, such as galaxy clusters, and act as stabilizing anchors for these massive systems.

Combined Influence:

• Gravitational strength at a node is the result of both filament length and density: which represents the density of the filament connecting to the i-th node.

Visualizing Tapering Effects

Tapering of Filaments:

• As filaments extend farther from a central node, their density decreases, and their gravitational influence tapers off.
• This tapering effect can be visualized as thinning strands extending outward, becoming less connected to distant nodes.

Illustrative Example:

• Imagine a spiderweb with a dense core and radiating strands that thin out toward the edges. The central mass dominates the gravitational dynamics, while the influence of outer strands diminishes with distance.

Stabilizing Role:

• Tapering ensures that local systems remain stable without being overwhelmed by distant gravitational influences. It creates a natural hierarchy of gravitational interactions, where nearby nodes exert the strongest pull.

Cosmic Web Perspective:

• The tapering of dark matter filaments explains the stability of the cosmic web, where galaxy clusters remain bound while the universe expands. Filaments create localized zones of influence interconnected by weaker, elongated strands.

This visualization supports the hypothesis that filament length and density are critical parameters in gravitational dynamics and structural stability.

3. Implications

3.1 Local Stability

Explaining Planetary Orbits and Galactic Clustering through Gravitational Averaging

Planetary Orbits:

Gravitational averaging provides a framework to understand how planets maintain stable orbits within a solar system. Each planet acts as a node influenced by:

• The dominant gravitational pull of the Sun as the central node.
• The minor gravitational effects of neighboring planets and dark matter filaments.

The weighted average of these influences creates the centripetal force required for stable orbital motion, ensuring that planets neither spiral into the Sun nor drift away.

Example of Earth’s Orbit:

Earth’s stable orbit arises from the combined effects of:

• The Sun’s overwhelming gravitational pull, transmitted directly through short filaments.
• Gravitational averaging with minor contributions from the Moon, neighboring planets, and dark matter filaments extending to the solar system’s edge.

Galactic Clustering:

At larger scales, gravitational averaging explains why galaxies form clusters and remain bound within them. Each galaxy acts as a node influenced by:

• The dense gravitational pull of nearby galaxies connected by short, thick filaments.
• The weaker, long-range influence of distant clusters connected by sparse filaments.

This averaging process stabilizes clusters, preventing galaxies from drifting apart despite the universe’s overall expansion.

Hierarchy of Gravitational Influence:

Within a galaxy cluster, local gravitational interactions dominate, creating tightly bound systems.

At the cluster’s edges, the tapering of filaments reduces gravitational influence, defining the cluster’s boundaries and connecting it to the broader cosmic web.

Conclusion:

Gravitational averaging unifies the dynamics of planetary orbits and galactic clustering by emphasizing the networked nature of gravitational influence. It shows how localized stability and large-scale coherence arise from the same principles, governed by the interplay of filament length, density, and connectivity.

3.2 Universal Connectivity

The image shows dark matter filaments spanning the cosmos and connected to “nodes” or bodies with different scales in a branch-like structure.

Cosmic Web as a Stabilizing Network

The cosmic web connects galaxy clusters, galaxies, and smaller-scale nodes through an intricate network of dark matter filaments. This network ensures that gravitational influence is not limited to local interactions but spans vast cosmic distances.

Long-Range Influence

While the gravitational pull from distant nodes diminishes with the square of the distance, the cumulative effect of countless nodes creates a stabilizing influence on large-scale structures. This prevents galaxies and clusters from drifting apart excessively during the universe’s expansion.

Dynamic Interaction

Universal connectivity ensures that gravitational forces between distant nodes remain balanced. This interplay is critical for maintaining the coherence of large-scale structures like galaxy clusters and superclusters within the ever-expanding universe.

Implications for Stability

The cosmic web serves as a unifying framework that harmonizes local gravitational interactions with the forces driving universal expansion. By maintaining a delicate balance between these opposing dynamics, it ensures the long-term stability and coherence of the universe’s structure, from individual galaxies to vast superclusters.

The previous image illustrates the concept of scale relativity, where the density of dark matter nodes determines both mass and gravitational force across different levels of abstraction — from atoms to humans to solar systems. It highlights how matter and gravity are interconnected through the dynamic network of dark matter filaments.

3.3 Dynamic Adaptation

Filaments as Adaptive Structures Responding to Changes in Node Mass and Connectivity

Dynamic Nature of Dark Matter Filaments

Dark matter filaments are not static entities; they adapt dynamically to the mass and distribution of the nodes they connect. As nodes (e.g., stars, galaxies, or galaxy clusters) gain or lose mass or change their positions due to cosmic events, the filaments adjust their properties, including length, density, and orientation, to maintain gravitational coherence across the cosmic web.

How Adaptation to Node Mass Could Work:

• When a node increases in mass (e.g., through accretion or merging with another node), its gravitational influence strengthens.
• Nearby filaments respond by increasing their density and tightening their connections to reflect the enhanced gravitational pull.
• Conversely, if a node loses mass (e.g., through stellar explosions or evaporation), the filaments weaken or stretch, redistributing their gravitational influence across connected nodes.

How Adjustments to Connectivity Could Work:

• As new nodes form or existing nodes move, filaments reconfigure to maintain connectivity within the cosmic web.
• New filaments can form to bridge gaps, while underutilized connections may dissipate or weaken over time.
• This adaptability prevents fragmentation of the cosmic web and ensures stability in both local systems (e.g., solar systems) and large-scale structures (e.g., galaxy clusters).

Stabilization Through Feedback Loops

The seemingly adaptive nature of filaments creates feedback loops that stabilize gravitational interactions:

• Local Adjustments: Changes in one part of the filament network are compensated by adjustments in neighboring regions, preventing sudden disruptions.
• Global Stability: The entire web works as a cohesive system, distributing gravitational forces efficiently even as individual nodes evolve over time.

Implications for Cosmic Evolution

This adaptive behavior has profound implications for understanding cosmic evolution:

• Galactic Formation: Filaments guide the flow of matter into forming galaxies, dynamically responding to the evolving mass of these systems.
• Cluster Coherence: The flexibility of filaments ensures that galaxy clusters remain bound despite changes in individual galaxies or the expansive forces of dark energy.

Conclusion
The observed adaptability of dark matter filaments underscores their critical role in maintaining the stability of the universe’s large-scale structure. By responding to changes in node mass and connectivity, these filaments act as self-regulating mechanisms that preserve the coherence and dynamism of the cosmic web.

4. Testing the Model

The image displays where one might see gravity “attractors” in a computer graphics simulation.

Blender Workflow

The video demonstrates a proof of concept which tries to communicate how gravitational averaging necessitates orbiting.

We propose simulating the effects of dark matter on a sphere representing the solar system’s outer boundary, composed of small rocky bodies. Each vertex of the sphere is connected to a central point, symbolizing the Sun. These connections generate an additive gravitational effect that increases the central gravitational pull of the solar system. At the same time, the collective gravitational influence of the small rocky bodies in the sphere creates a counteracting pull. The tessellation density of the sphere determines the mass distribution required to achieve gravitational equilibrium, enabling stable celestial body orbits within the solar system.

Python Script for an Orbiting Sphere

If you prefer scripting, here’s a snippet to automate the setup. This is a simple setup with 3 spheres exhibiting equilibrium (translate one of the large spheres to see the effect):

import bpy
import random

# Delete all objects in the scene
bpy.ops.object.select_all(action='SELECT')
bpy.ops.object.delete(use_global=False)

# Function to create a sphere with mass and rigid body settings
def create_sphere(name, location, scale, mass):
    bpy.ops.mesh.primitive_uv_sphere_add(radius=1, location=location)
    sphere = bpy.context.object
    sphere.name = name
    sphere.scale = scale

    # Add rigid body physics
    bpy.ops.rigidbody.object_add()
    sphere.rigid_body.type = 'ACTIVE'
    sphere.rigid_body.mass = mass
    sphere.rigid_body.friction = 0.5
    sphere.rigid_body.restitution = 0.3

    return sphere

# Function to create a large attractor with a ring and force field
# Attractor is set to be static

def create_attractor(name, location, scale, mass, force_strength):
    bpy.ops.mesh.primitive_uv_sphere_add(radius=1, location=location)
    attractor = bpy.context.object
    attractor.name = name
    attractor.scale = scale

    # Add rigid body physics
    bpy.ops.rigidbody.object_add()
    attractor.rigid_body.type = 'PASSIVE'  # Make the attractor static
    attractor.rigid_body.mass = mass
    attractor.rigid_body.friction = 0.5
    attractor.rigid_body.restitution = 0.3

    # Add a ring to indicate the attractor
    bpy.ops.mesh.primitive_circle_add(radius=scale[0] * 1.5, location=location)
    ring = bpy.context.object
    ring.name = f"{name}_Ring"
    ring.scale = (1, 1, 1)
    ring.hide_render = True  # Optional: Hide ring in render

    # Add a force field to simulate gravitational pull
    bpy.ops.object.effector_add(type='FORCE', location=location)
    force_field = bpy.context.object
    force_field.name = f"{name}_Force"
    force_field.field.type = 'FORCE'
    force_field.field.strength = force_strength
    force_field.field.falloff_type = 'SPHERE'

    return attractor

# Create large attractors with Sun-like force, rings, and force fields
attractors = [
    create_attractor("Attractor1", location=(10, 10, 0), scale=(2, 2, 2), mass=50.0, force_strength=-400),
    create_attractor("Attractor2", location=(-10, -10, 0), scale=(2, 2, 2), mass=50.0, force_strength=-400),
    create_attractor("Attractor3", location=(10, -10, 0), scale=(2, 2, 2), mass=50.0, force_strength=-400),
    create_attractor("Attractor4", location=(-10, 10, 0), scale=(2, 2, 2), mass=50.0, force_strength=-400),
    create_attractor("Attractor5", location=(0, 15, 0), scale=(2, 2, 2), mass=50.0, force_strength=-400),
]

# Create smaller spheres with varying sizes, masses, and random initial positions
spheres = []
for i in range(3):
    loc = (random.uniform(-5, 5), random.uniform(-5, 5), random.uniform(-5, 5))
    scale = (random.uniform(0.5, 1.0), random.uniform(0.5, 1.0), random.uniform(0.5, 1.0))
    mass = random.uniform(1.0, 3.0)
    sphere = create_sphere(f"Sphere{i+1}", location=loc, scale=scale, mass=mass)
    spheres.append(sphere)

# Add some randomness to the initial velocities of the spheres
def set_initial_velocity(sphere, velocity):
    sphere.rigid_body.linear_velocity = velocity

for sphere in spheres:
    velocity = (random.uniform(-2, 2), random.uniform(-2, 2), random.uniform(-2, 2))
    set_initial_velocity(sphere, velocity)

# Set up the simulation environment
bpy.context.scene.rigidbody_world.time_scale = 1.0
bpy.context.scene.rigidbody_world.steps_per_second = 120
bpy.context.scene.rigidbody_world.solver_iterations = 10

# Set the end frame of the animation
bpy.context.scene.frame_end = 500

print("Simulation setup complete. Run the animation to see the results.")

The image displays an illustration of a sparse data set to simulate gravity in a small spherical solar system.

5. Broader Applications

1. Enhancing Astrophysics: Better Models for Dark Matter Distribution

Gravitational averaging offers a unified framework for analyzing dark matter distribution and its role in cosmic stability. This could refine predictive models for galaxy formation and gravitational lensing effects (Springel et al., 2005; Schaye et al., 2015).

Predictive Power:
Incorporating gravitational averaging into simulations allows researchers to predict how dark matter filaments influence galactic formation and evolution. This can help identify regions of high dark matter density that are critical for understanding galaxy clustering and cosmic web dynamics.

Resolving Discrepancies:
Current models often struggle to reconcile dark matter behavior across different scales. Gravitational averaging provides a unified explanation that bridges local gravitational effects (e.g., planetary orbits) and large-scale phenomena (e.g., galaxy clusters).

Mapping Dark Matter:
This theory supports more accurate mapping of dark matter distributions by considering filament density and tapering effects. Observational data, such as that from gravitational lensing or cosmic microwave background studies, could be reanalyzed with this framework to refine our understanding of the dark matter network.

2. Advancing Computer Graphics: Simulations of Realistic Gravitational Dynamics

Gravitational averaging principles can inspire the next generation of computer graphics algorithms, enabling more realistic and scientifically accurate simulations of gravitational interactions.

Physics-Based Simulations:
By modeling nodes, filaments, and their adaptive behavior, computer graphics engines can simulate complex gravitational systems with unprecedented fidelity. This is particularly relevant for visualizing cosmic phenomena in films, games, and educational content.

Efficient Particle Systems:
The filament length principle and adaptive connectivity can optimize particle-based simulations, reducing computational overhead while maintaining realism. Artists and developers could use these techniques to simulate dense, dynamic systems like planetary formation or galaxy collisions.

Educational Tools:
Visualizing the cosmic web and its interactions with gravitational averaging could create powerful educational tools. Students and researchers alike could explore these phenomena interactively, gaining deeper insights into cosmic dynamics.

By modeling gravitational dynamics based on the filament length principle, simulations in computer graphics could achieve unparalleled accuracy. Applications include films, games, and educational tools visualizing the cosmic web (Dolag et al., 2009).

The Laws of Light and Gravity: Foundations for a New Era of Cosmic Exploration

1. The Law of Gravitational Averaging

Gravity is not the force of a single body but the averaged influence of all connected masses, modulated by their relative distances and filament strengths.

• Significance: Explains how multi-body systems achieve stability, unifying local gravitational interactions with large-scale dynamics.
• Example: Simulations of planetary formation showing equilibrium zones formed through averaging.

2. The Filamentary Stability Principle

Dark matter filaments act as gravitational pathways, stabilizing celestial structures by dynamically distributing forces across connected nodes.

• Significance: Provides a mechanism for the cosmic web’s role in galaxy clustering and universal structure.
• Example: Filaments connecting galaxy clusters create a balanced gravitational network.

3. The Goldilocks Zone of Gravitational Balance

Stable orbits and cosmic equilibrium arise from a balance between gravitational forces within and beyond a system, constrained by the scale and mass of the system.

• Significance: Establishes conditions for the stability of planets, stars, and systems in the universe.
• Example: Ships in gravitational averaging simulations stabilizing in specific zones.

4. The Dark Matter Equilibrium Hypothesis

Dark matter leverages gravity to organize masses into equilibrium, stabilizing cosmic structures through dynamic interactions.

• Significance: Suggests dark matter is not passive but actively shapes universal stability.
• Example: Dark matter filaments acting as conduits to balance gravitational forces.

5. The Connectivity Strength Principle

The strength and influence of gravitational forces are proportional to the density and length of dark matter filaments connecting celestial bodies.

• Significance: Explains the tapering effects of gravitational pull and the structure of the cosmic web.
• Example: Filaments thinning as they extend farther from central nodes, reducing gravitational influence.

6. Law of Decentralized Swarm Coordination

Drone swarms leveraging blockchain technology achieve efficient, secure, and scalable coordination. Each drone acts as a node in a blockchain network, ensuring tamper-proof communication and dynamic task allocation through smart contracts. This decentralized approach allows real-time swarm reconfiguration, resource optimization, and resilience, enabling applications in logistics, defense, disaster relief, and space exploration.

7. The Volumetric Modularity Principle

Systems designed with modular, volumetric configurations can leverage gravitational and energetic forces for dynamic adaptation and stability.

• Significance: Enables the design of systems capable of shape-shifting and distributed functionality.
• Example: Modular spacecraft configurations optimized for propulsion and equilibrium.

8. Law of Distributed Scalability and Reconstitution

A distributed system’s scalability is limited only by the availability of resources, the energy efficiency of transportation to orbit, and manufacturing time. Modular components launched from multiple locations enable decentralized deployment and self-assembly in space, supported by in-situ energy harvesting and advanced propulsion technologies.

9. Hypothesis of Modular Reconstitution in Space

Given a modular, distributed architecture, individual components launched from multiple global locations can autonomously reconstitute into a cohesive system in orbit. This process is enabled by:

• Efficient launch strategies (e.g., reusable rockets and optimized payloads).
• In-space energy harvesting (e.g., solar arrays and in-situ fuel production).
• Orbital assembly platforms leveraging robotic and AI systems. This approach ensures scalability, sustained operation, and rapid deployment with minimized energy costs.

10. Law of Modular Evolution in Distributed Systems

A modular system equipped with onboard manufacturing capabilities can evolve and adapt post-deployment, eliminating the need for perfect design prior to launch and ensuring continuous improvement and scalability.

11. Hypothesis of In-System Manufacturing and Upgrades

By integrating manufacturing systems into distributed hubs, a modular fleet can autonomously produce, repair, and upgrade components in real time, allowing for infinite adaptability and eliminating the constraints of pre-deployment perfection.

• Eliminates Perfect Design Dependency: Systems can adapt to unforeseen challenges and changing mission requirements after deployment.
• Infinite Scalability: New modules and components can be manufactured directly in space, leveraging resources harvested from celestial bodies or the Arc’s energy systems.
• Increased Longevity: Continuous upgrades and repairs extend the operational lifespan of the fleet, reducing the need for Earth-based resupply missions.
• Localized Problem Solving: Hubs can address specific regional or mission-critical needs without requiring centralized intervention.

12. Law of Gravitational Mass Stabilization

In high-velocity gravitational slingshot systems, total mass must be proportional to the expected slingshot velocity of the inbound system. Increasing system mass dynamically can stabilize forces, prevent structural failure, and ensure controlled deceleration.

13. Hypothesis of Holographic Gravitational Mapping

By applying the Holographic Principle to computer graphics, volumetric datasets encoding gravitational data can be compressed and represented on a 2D texture. This enables ultra-detailed simulations of gravitational systems with fidelity limited only by texture resolution, paving the way for scalable, data-rich representations of universal dynamics.

14. Law of Holographic Data Encoding for Gravitational Simulations

Volumetric gravitational data can be encoded within 2D surfaces, where each pixel stores multi-dimensional gravitational properties. This allows for the compression, simulation, and manipulation of complex gravitational systems at scales ranging from atomic to cosmic, mimicking the universe’s underlying holographic nature (Hooft 1993).

Key Components

1. 2D Encoding of 3D Gravitational Data:

• Each pixel in the texture map encodes gravitational metrics like mass, velocity, density, and direction.
• Texture resolution dictates the fidelity of the simulation, providing flexibility in computational demand.

Scalable Fidelity

Lens Effect:

• Focus simulations on specific scales (e.g., molecular, planetary, galactic) by “lensing” resolution.
• Enables multi-scale simulations where universal laws operate consistently across scales.

Data Compression:

• Encodes complex 3D interactions into 2D surfaces using holographic principles, drastically reducing storage and computational overhead.

Simulative Realism:

• Mimics the behavior of the universe by leveraging gravitational fidelity.
• Ideal for studying dark matter, gravitational lensing, and cosmic web formation.

Applications

1. Super Data-Rich Simulations:

• Simulate planetary systems, black holes, and cosmic structures with unprecedented detail.
• Run simulations at multiple scales (e.g., atomic vs. galactic) using the same encoding framework.

1. AI and Machine Learning: Use holographic textures as training datasets for AI models to understand and predict gravitational interactions.
2. Game and Film Industry: Enhance volumetric effects and realistic gravitational dynamics in video games and movies.
3. Space Exploration: Simulate trajectories, gravitational slingshots, and asteroid deflections with extreme precision for mission planning.

Holographic Mapping in Practice

Texture Encoding:

• Gravitational fields and properties are stored in a high-resolution 2D texture using multi-channel encoding (e.g., RGBA channels for density, velocity, and directional vectors).

Real-Time Simulation:

• Graphics engines process 2D textures to calculate gravitational effects on 3D objects in real time.
• Efficient for interactive applications, such as VR or AR simulations.

Data Compression Framework: Use lossless or near-lossless compression to maximize detail while minimizing storage.

Why This Matters

• Efficiency: Encoding volumetric data in 2D reduces the cost and complexity of high-fidelity simulations.
• Universal Modeling: Mimics the universe’s own compression principles, enabling realistic simulations at any scale.
• Scalability: Makes data-heavy applications accessible for industries ranging from entertainment to astrophysics.

15. Hypothesis: Oscillatory Resonance in Dark Matter Filaments

Statement:
Biological wing flapping in bees generates oscillatory forces that interact with dark matter filaments, creating a resonant effect that stabilizes motion, enhances propulsion efficiency, and reduces environmental drag.

Key Elements of the Hypothesis:

Biological Insight:

• Bees and similar organisms demonstrate extraordinary energy efficiency in long-distance migrations and swarming behaviors.
• This efficiency hints at an unseen stabilizing mechanism that may interact with their oscillatory wing dynamics.

Cosmic Connection:

• Dark matter filaments, theorized to act as gravitational highways, could provide this stabilizing influence by interacting with the oscillatory forces of wing flapping.

Implications:

• Explains natural flight efficiency and stability in swarming behaviors.
• Provides a framework for biomimetic applications in drones, spacecraft propulsion, and energy-efficient travel systems.

Testable Predictions:

• High-frequency oscillatory motion in engineered systems should produce measurable stabilization effects when interacting with dark matter filaments or analogous simulated systems.
• Bees or small drones in controlled environments (e.g., vacuums or low-gravity chambers) might exhibit unique flight characteristics due to unopposed interactions with unseen forces.

Applications:

• Drone Technology: Development of filament-sensitive propulsion systems for swarming drones.
• Space Exploration: Designing spacecraft with oscillatory propulsion for efficient filament tunneling.
• Energy Efficiency: Leveraging oscillatory motion to reduce energy expenditure in biomimetic systems.

16. Hypothesis: Diamond-Assisted Precision Monitoring and Treatment System

Statement:
A diamond-based therapeutic system can utilize its high thermal conductivity, optical clarity, and durability to deliver and monitor precise energy-based treatments for harmful cells, while integrating advanced imaging and AI-driven feedback for adaptive control and real-time progress tracking.

Key Insights:

Energy Delivery:

• Diamonds focus and stabilize laser-induced oscillations, ensuring energy is concentrated on targeted cells without damaging surrounding tissue.

Monitoring Mechanisms:

• Embedded sensors measure thermal, pressure, and vibrational effects.
• Real-time imaging (e.g., OCT, ultrasound) captures structural changes.
• Biomarkers and cfDNA levels track biochemical responses to treatment.

AI Optimization:

• Machine learning algorithms analyze imaging and sensor data, adjusting energy delivery patterns for maximum efficacy.

Desired Outcomes:

• Selective Targeting: Harmful cells will exhibit measurable disruption under diamond-assisted oscillatory treatments, ensuring precise therapeutic impact.
• Minimal Damage: Surrounding healthy tissue will remain unaffected, as validated by advanced imaging and biomarker analysis.
• Adaptive Efficiency: AI-driven feedback loops will dynamically optimize energy delivery in real time, significantly improving treatment outcomes over traditional methods.
• Cellular Filament Tunneling: Gravitational averaging principles can be harnessed at the cellular level to achieve targeted manipulation, offering a novel therapeutic approach.

17. Law of Gravity-Induced Filament Curvature

Statement:
Dark matter filaments connecting nodes exhibit natural curvature under the influence of gravitational forces, reflecting the dynamic interplay of mass and gravitational fields. This curvature, or “gravity drooping,” represents an optimized path of least resistance, balancing forces across scales.

Key Principles:

Curvature as a Stabilizing Mechanism:

• Filament curvature enhances the stability of connected systems, ensuring equilibrium between gravitational pull and mass distribution.
• This effect scales from microscopic interactions (e.g., cellular systems) to cosmic structures (e.g., the cosmic web).

Efficiency of Curved Paths:

• Curved filaments reduce energy expenditure in systems, allowing forces to distribute dynamically and efficiently.
• The drooping effect optimizes connectivity by adapting to local gravitational conditions.

Multi-Scale Universality:

• Gravity-induced curvature is observed at all levels, from biological nodes (e.g., cells) to celestial systems (e.g., galaxies), suggesting a universal design principle.

Hypothesis:

“Gravity drooping” in filament networks provides both stability and efficiency by allowing nodes to adapt dynamically to gravitational forces. This principle could be applied to distributed systems, biological modeling, and astrophysical simulations.

18. Principle of Lensing Fidelity

Statement:
Lensing Fidelity defines the density and level of detail in dark matter filament visualizations, reflecting the dynamic scaling of filaments based on the observer’s focus or abstraction level.

Key Aspects:

Density Scaling:

• The number and thickness of filaments increase with higher fidelity, emphasizing fine-grained connections between nodes.
• Lower fidelity reduces filament density, focusing on macro-scale structures for clarity and simplicity.

Dynamic Adaptation:

• The principle adapts to the lensing scale, from universal (cosmic web) to human (room or outdoor settings), while maintaining proportional relationships and curvature.

Applications:

• Scientific Visualization: Communicate the complexity of gravitational or dark matter systems at various scales.
• Simulation Models: Dynamically adjust filament density for efficient computation and realistic renderings.
• Education: Provide scalable representations for different levels of scientific understanding.

19. Cosmic Hypernova Structure Theory

The Cosmic Hypernova Structure Theory proposes that hypernovae, among the most energetic events in the universe, act as crucial waypoints in the formation and evolution of galactic and cosmic structures. These massive explosions not only redistribute matter and energy but also play a pivotal role in shaping the cosmic web and enriching galaxies with the building blocks of life.

Hypernovae as Cosmic Nodes:

• Hypernovae serve as central points of energy release, ejecting material that aligns with or reinforces the filamentary structure of the cosmic web.

Filamentary Connections:

• The interaction of hypernova ejecta with dark matter scaffolding and gravitational forces shapes intricate filaments, connecting galaxies, quasars, and nebulae.

Foundations of Galactic Evolution:

• The heavy elements expelled by hypernovae are essential for star formation, planet creation, and life, while their gravitational influence organizes surrounding matter into larger structures.

A Cycle of Creation and Connection:

• Rather than being destructive endpoints, hypernovae are integral to a cycle of cosmic renewal, acting as waypoints that connect and sustain the growth of the universe at all scales.

20. The Law of Harmonic Gravitational Sorting

Thesis:
Planetary ring systems, such as Saturn’s rings, are the result of harmonic gravitational sorting, a process in which particles of varying mass self-organize into stable, repeating patterns under the influence of a planet’s gravitational field. This sorting reflects an equilibrium shaped by local dynamics and supported by the larger gravitational framework established by dark matter.

Mass-Based Sorting:

• Particles with similar mass and composition cluster together, creating distinct, color-coded sedimentary patterns that align with their gravitational interactions.

Harmonic Banding:

• Resonances within the gravitational field, amplified by interactions with planetary moons, produce repeating bands that reflect the dynamic balance of forces.

Dark Matter Influence:

While dark matter filaments do not directly form planetary rings, they provide the gravitational scaffolding that supports baryonic matter’s harmonic sorting, allowing these intricate structures to emerge.

Universal Implications:

• This law extends to other planetary rings, protoplanetary disks, and potentially larger cosmic structures, where dark matter creates the framework for baryonic matter to self-organize into resonant, patterned systems.

21. The Law of Gradient Faceted Spherical Design

Statement:
A spherical diamond’s surface can be optimally designed by blending faceted and smooth regions through a gradient mask along the vertical axis (t-direction). This law ensures the seamless integration of optical functionality (faceted precision) and rotational or mechanical efficiency (smooth surface) for advanced applications.

Implications

Optical Applications:

• The faceted top ensures precision light refraction for imaging or laser targeting.
• The smooth bottom provides rotational or mechanical stability.

Design Scalability:

• The gradient mask can be adjusted for different sizes or specific applications.

Aesthetic and Functional Harmony:

• Combines beauty with precision, making it ideal for scientific, medical, and artistic uses.

22. Law of Gravitational Equilibrium Mapping for Precision Landings

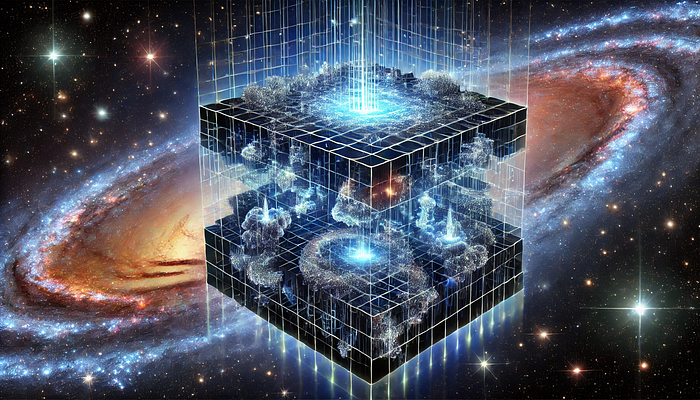
Statement:
A vessel can achieve efficient and precise landings by reverse-engineering gravitational nodes and edges from volumetric LIDAR data. By leveraging these structures, the vessel’s thrusters dynamically counterbalance forces, aligning with local equilibrium zones influenced by terrain and dark matter filaments, resulting in minimal energy expenditure and maximum stability (Bardeen et. al 1972).

Key Principles

LIDAR-Derived Geometry as Input:

• The terrain’s volumetric LIDAR data serves as the foundation for identifying gravitational nodes and edges.
• The system analyzes mass distribution and structural relationships in real-time.

Gravitational Node and Edge Mapping:

• Gravitational nodes are points of stability derived from mass concentrations.
• Edges represent the predicted pathways of gravitational influence, analogous to dark matter filaments.

Dynamic Thruster Counterbalancing:

• Thrusters adjust dynamically based on the predicted equilibrium map, ensuring rapid and precise alignment with stable zones.

Energy Efficiency Through Equilibrium:

• The system minimizes energy consumption by reducing thruster overcorrections, leveraging gravitational equilibrium zones as natural stabilizers.

Implications

Landing Precision:

• By aligning with equilibrium zones, landings become smoother and more accurate, even on irregular terrain.

Energy Conservation:

• Thrusters engage minimally, reducing fuel consumption and operational costs.

Scalability:

• The system adapts to different environments (e.g., planetary surfaces, asteroids, moons) using LIDAR as the universal input.

Applications

Planetary Landings:

• Enables rapid and efficient spacecraft landings on uneven or unstable surfaces.

Asteroid Docking:

• Maps gravitational nodes on small celestial bodies to guide precise docking maneuvers.

Emergency Landings:

• Real-time node and edge mapping ensures safe and controlled landings in dynamic conditions.

Examples of Implementation

Simulations:

• Use Blender to simulate landings with LIDAR-derived terrains and gravitational mapping.

Prototyping:

• Test the system with drones equipped with LIDAR and AI-guided thrusters on scaled-down terrains.

23. Hypothesis: Gravitational Averaging as the Basis for G-Force Resistance

Statement:
G-force experienced by an object or individual during acceleration, deceleration, or maneuvering arises as a measurable effect of deviations from gravitational averaging equilibrium. This resistance is proportional to the extent to which the object “fights” the local gravitational averaging equilibrium, defined by nodes, edges, and filamentary structures.

Key Principles

Gravitational Averaging Equilibrium:

• In any system, gravitational averaging defines a stable zone of balanced forces, where a body experiences minimal resistance (e.g., hovering at a gravitational node or traveling along a gravitational filament).

Deviations from Equilibrium:

• Accelerating, decelerating, or maneuvering across gravitational edges (or perpendicular to filaments) disrupts this balance, leading to a measurable inertial resistance — commonly experienced as G-force.

G-Force as a Result of Disruption:

• The magnitude of G-force experienced is proportional to the extent of deviation from the equilibrium forces defined by gravitational averaging.

Predictions

Low G-Force in Equilibrium:

• A craft traveling along gravitational nodes or filaments will experience minimal G-force due to alignment with the equilibrium forces.

High G-Force in Deviations:

• Abrupt acceleration, sharp turns, or deviations from gravitational nodes will result in higher G-force due to greater disruption of equilibrium.

Energy Efficiency:

• Navigation strategies aligned with gravitational averaging principles will consume less energy, as they minimize resistance forces.

Testing the Hypothesis

Simulations:

• Use software (e.g., Blender, MATLAB) to model a system of gravitational nodes and filaments.
• Simulate the motion of objects across equilibrium zones and measure the inertial forces generated.

Experimental Prototypes:

• Equip a drone with sensors and algorithms to map gravitational equilibrium zones using real-world LIDAR data.
• Measure G-forces as the drone navigates along versus across these predicted zones.

Real-World Data:

• Analyze high-speed vehicles or spacecraft to correlate G-forces with deviations from optimal trajectories (e.g., smooth arcs vs. sharp turns).

The video demonstrates spheres under the influence of a complex gravitational field and equilibrium is demonstrated via gravitational averaging.

24. The Law of Gravitational Averaging for Relativistic Motion

Statement:
Gravitational averaging provides a framework for understanding motion and inertial resistance (e.g., G-force) as the result of deviations from gravitational equilibrium. This equilibrium is determined by a dynamic web of gravitational nodes, edges, and filaments, which define the most efficient pathways for motion through spacetime.

Core Principles

1. Gravitational Filaments as Dynamic Geodesics
   Gravitational filaments, formed by local mass distributions and dark matter influences, act as natural pathways of least resistance. These filaments align with the geodesics described by general relativity, providing an optimized route for minimizing energy consumption and G-force.
2. Deviations Cause Resistance
   When a body moves along a filament, it experiences minimal resistance due to alignment with the gravitational averaging equilibrium. Deviations from these filaments — whether due to acceleration, deceleration, or lateral motion — generate inertial forces, perceived as G-force.
3. Dynamic Adaptation of Filaments
   Gravitational nodes and filaments dynamically shift in response to changes in local mass and energy distributions, maintaining equilibrium across scales. This adaptability ensures stability in complex systems, from planetary orbits to spacecraft navigation.
4. Relativistic Integration
   Time dilation and length contraction, as predicted by special relativity, naturally occur within gravitational filament dynamics. Dense filaments correlate with slower passage of time, offering a framework to predict and leverage relativistic effects in motion.

Testable Predictions

1. Energy Efficiency
   Bodies traveling along gravitational filaments will require less energy for propulsion compared to those moving perpendicular to or away from filaments.
2. Reduced G-Force
   Motion along gravitational filaments will result in minimal G-force, whereas deviations will cause higher resistance proportional to the degree of disruption.
3. Time Dilation Correlation
   Dense gravitational filaments, where averaging forces are strongest, will exhibit greater time dilation, aligning with predictions of general relativity.

Applications

1. Space Travel
   Gravitational filament mapping could guide spacecraft along efficient trajectories, reducing energy consumption and minimizing stress on passengers and structural systems.
2. Vehicle Dynamics
   Aircraft, cars, and ships could optimize their paths to align with local gravitational averaging principles, improving fuel efficiency and reducing wear.
3. Scientific Insights
   The law of gravitational averaging could refine our understanding of how local and universal gravitational systems interact, providing new tools for exploring the universe.

25. Hypothesis: Filament-Centered Travel as a Relativistic Wormhole

Statement:
Traveling through the center of a gravitational filament at relativistic speeds generates a wormhole-like effect, where spatial traversal is rapid, but onboard time dilation ensures precise and efficient decision-making. This temporal buffer allows vessels to adjust trajectories and avoid catastrophic events, effectively blending relativity with practical navigation (Einstein et. al 1935).

Conclusion

The gravitational averaging theory bridges theoretical astrophysics and practical applications in computer graphics. By enhancing our understanding of dark matter distribution and enabling more accurate simulations, this framework has the potential to reshape both scientific research and the way we visualize the universe.

References

Bardeen, J. M., Press, W. H., & Teukolsky, S. A. 1972. “Rotating Black Holes: Locally Nonrotating Frames, Energy Extraction, and Scalar Synchrotron Radiation.” The Astrophysical Journal, 178, 347.

Bond, J. R., Kofman, L., & Pogosyan, D. 1996. “How Filaments of Galaxies Are Woven into the Cosmic Web.” Nature, vol. 380, pp. 603–606. DOI: 10.1038/380603a0.

Dietrich, J. P., et al. 2012. “A Filament of Dark Matter between Two Clusters of Galaxies.” Nature, vol. 487, pp. 202–204. DOI: 10.1038/nature11224.

Dolag, K., Borgani, S., Murante, G., & Springel, V. 2009. “Substructures in Hydrodynamical Cluster Simulations.” Monthly Notices of the Royal Astronomical Society, vol. 399, pp. 497–514.
DOI: 10.1111/j.1365–2966.2009.15241.x.

Einstein, A., & Rosen, N. 1935. “The Particle Problem in the General Theory of Relativity.” Physical Review, 48, 73.

Fallat, Rich. 2024. “Mapping the Universe: How Dark Matter Mirrors Computer Graphics and Could Impact Cosmology.” In medium. December: https://medium.com/@richfallatjrshop/dark-matter-573cddff7361.

Fallat, Rich. 2025. “Post (video).” In Instagram. https://www.instagram.com/reel/DEhcQ8FJIU4/?utm_source=ig_web_button_share_sheet&igsh=MzRlODBiNWFlZA==.

Forero-Romero, J. E., et al. 2009. “A Dynamical Classification of the Cosmic Web.” Monthly Notices of the Royal Astronomical Society, vol. 396, pp. 1815–1824. DOI: 10.1111/j.1365–2966.2009.14885.x.

Hooft, G. (1993). “Dimensional Reduction in Quantum Gravity.” arXiv:gr-qc/9310026.

Navarro, J. F., Frenk, C. S., & White, S. D. M. 1996. “The Structure of Cold Dark Matter Halos.” The Astrophysical Journal, vol. 462, 1996, pp. 563–575. DOI: 10.1086/177173.

Peebles, P. J. E. 1980. “The Large-Scale Structure of the Universe.” Princeton University Press.

Saplakoglu, Yasemin. 2019. “Dark ‘Half-Magnets’ from the Sun Could Be Streaming Through Us Every Day.” In space.com: News. Accessed (1/1/25): https://www.space.com/electron-microscope-could-detect-dark-half-magnets.html.

Schaye, J., et al. 2015. “The EAGLE Project: Simulating the Evolution and Assembly of Galaxies and Their Environments.” Monthly Notices of the Royal Astronomical Society, vol. 446, pp. 521–554. DOI: 10.1093/mnras/stu2058.

Springel, V. 2005. “The Cosmological Simulation Code GADGET-2.” Monthly Notices of the Royal Astronomical Society, vol. 364, pp. 1105–1134. DOI: 10.1111/j.1365–2966.2005.09655.x.

Springel, V., et al. (2005). “Simulations of the Formation, Evolution, and Clustering of Galaxies and Dark Matter.” Nature, 435, 629.

Springel, V., et al. N.A. “Simulations of the Formation, Evolution, and Clustering of Galaxies and Dark Matter.” Nature, vol. 435, 2005, pp. 629–636. DOI: 10.1038/nature03597.

Vogelsberger, M., et al. 2014. “Properties of Galaxies Reproduced by a Hydrodynamic Simulation.” Nature, vol. 509, pp. 177–182. DOI: 10.1038/nature13316.

Copyright Notice
© 2025 Los Angeles Mercantile. All rights reserved.
This article, including all concepts, visuals, and intellectual property contained herein, is the exclusive property of Los Angeles Mercantile. Unauthorized use, reproduction, or distribution is strictly prohibited.